Tag: statistics

scientists aren’t dumb; statistics is hard

There’s a feature article in the new issue of Science News on the failure of science “to face the shortcomings of statistics”. The author, Tom Siegfried, argues that many scientific results shouldn’t be believed because they depend on faulty statistical practices:

Even when performed correctly, statistical tests are widely misunderstood and frequently misinterpreted. As a result, countless conclusions in the scientific literature are erroneous, and tests of medical dangers or treatments are often contradictory and confusing.

I have mixed feelings about the article. It’s hard to disagree with the basic idea that many scientific results are the results of statistical malpractice and/or misfortune. And Siegfried generally provides lucid explanations of some common statistical pitfalls when he sticks to the descriptive side of things. For instance, he gives nice accounts of Bayesian inference, of the multiple comparisons problem, and of the distinction between statistical significance and clinical/practical significance. And he nicely articulates what’s wrong with one of the most common (mis)interpretations of p values:

Correctly phrased, experimental data yielding a P value of .05 means that there is only a 5 percent chance of obtaining the observed (or more extreme) result if no real effect exists (that is, if the no-difference hypothesis is correct). But many explanations mangle the subtleties in that definition. A recent popular book on issues involving science, for example, states a commonly held misperception about the meaning of statistical significance at the .05 level: “This means that it is 95 percent certain that the observed difference between groups, or sets of samples, is real and could not have arisen by chance.“

So as a laundry list of common statistical pitfalls, it works quite nicely.

What I don’t really like about the article is that it seems to lay the blame squarely on the use of statistics to do science, rather than the way statistical analysis tends to be performed. That’s to say, a lay person reading the article could well come away with the impression that the very problem with science is that it relies on statistics. As opposed to the much more reasonable conclusion that science is hard, and statistics is hard, and ensuring that your work sits at the intersection of good science and good statistical practice is even harder. Siegfried all but implies that scientists are silly to base their conclusions on statistical inference. For instance:

It’s science’s dirtiest secret: The “scientific method“ of testing hypotheses by statistical analysis stands on a flimsy foundation. Statistical tests are supposed to guide scientists in judging whether an experimental result reflects some real effect or is merely a random fluke, but the standard methods mix mutually inconsistent philosophies and offer no meaningful basis for making such decisions.

Or:

Experts in the math of probability and statistics are well aware of these problems and have for decades expressed concern about them in major journals. Over the years, hundreds of published papers have warned that science’s love affair with statistics has spawned countless illegitimate findings. In fact, if you believe what you read in the scientific literature, you shouldn’t believe what you read in the scientific literature.

The problem is that there isn’t really any viable alternative to the “love affair with statistics”. Presumably Siegfried doesn’t think (most) scientists ought to be doing qualitative research; so the choice isn’t between statistics and no statistics, it’s between good and bad statistics.

In that sense, the tone of a lot of the article is pretty condescending: it comes off more like Siegfried saying “boy, scientists sure are dumb” and less like the more accurate observation that doing statistics is really hard, and it’s not surprising that even very smart people mess up frequently.

What makes it worse is that Siegfried slips up on a couple of basic points himself, and says some demonstrably false things in a couple of places. For instance, he explains failures to replicate genetic findings this way:

Nowhere are the problems with statistics more blatant than in studies of genetic influences on disease. In 2007, for instance, researchers combing the medical literature found numerous studies linking a total of 85 genetic variants in 70 different genes to acute coronary syndrome, a cluster of heart problems. When the researchers compared genetic tests of 811 patients that had the syndrome with a group of 650 (matched for sex and age) that didn’t, only one of the suspect gene variants turned up substantially more often in those with the syndrome — a number to be expected by chance.

“Our null results provide no support for the hypothesis that any of the 85 genetic variants tested is a susceptibility factor“ for the syndrome, the researchers reported in the Journal of the American Medical Association.

How could so many studies be wrong? Because their conclusions relied on “statistical significance,“ a concept at the heart of the mathematical analysis of modern scientific experiments.

This is wrong for at least two reasons. One is that, to believe the JAMA study Siegfried is referring to, and disbelieve the results of all 85 previously reported findings, you have to accept the null hypothesis, which is one of the very same errors Siegfried is supposed to be warning us against. In fact, you have to accept the null hypothesis 85 times. In the JAMA paper, the authors are careful to note that it’s possible the actual effects were simply overstated in the original studies, and that at least some of the original findings might still hold under more restrictive conditions. The conclusion that there really is no effect whatsoever is almost never warranted, because you rarely have enough power to rule out even very small effects. But Siegfried offers no such qualifiers; instead, he happily accepts 85 null hypotheses in support of his own argument.

The other issue is that it isn’t really the reliance on statistical significance that causes replication failures; it’s usually the use of excessively liberal statistical criteria. The problem has very little to do with the hypothesis testing framework per se. To see this, consider that if researchers always used a criterion of p < .0000001 instead of the conventional p < .05, there would almost never be any replication failures (because there would almost never be any statistically significant findings, period). So the problem is not so much with the classical hypothesis testing framework as with the choices many researchers make about how to set thresholds within that framework. (That’s not to say that there aren’t any problems associated with frequentist statistics, just that this isn’t really a fair one.)

Anyway, Siegfried’s explanations of the pitfalls of statistical significance then leads him to make what has to be hands-down the silliest statement in the article:

But in fact, there’s no logical basis for using a P value from a single study to draw any conclusion. If the chance of a fluke is less than 5 percent, two possible conclusions remain: There is a real effect, or the result is an improbable fluke. Fisher’s method offers no way to know which is which. On the other hand, if a study finds no statistically significant effect, that doesn’t prove anything, either. Perhaps the effect doesn’t exist, or maybe the statistical test wasn’t powerful enough to detect a small but real effect.

If you take this statement at face value, you should conclude there’s no point in doing statistical analysis, period. No matter what statistical procedure you use, you’re never going to know for cross-your-heart-hope-to-die sure that your conclusions are warranted. After all, you’re always going to have the same two possibilities: either the effect is real, or it’s not (or, if you prefer to frame the problem in terms of magnitude, either the effect is about as big as you think it is, or it’s very different in size). The same exact conclusion goes through if you take a threshold of p < .001 instead of one of p < .05: the effect can still be a spurious and improbable fluke. And it also goes through if you have twelve replications instead of just one positive finding: you could still be wrong (and people have been wrong). So saying that “two possible conclusions remain” isn’t offering any deep insight; it’s utterly vacuous.

The reason scientists use a conventional threshold of p < .05 when evaluating results isn’t because we think it gives us some magical certainty into whether a finding is “real” or not; it’s because it feels like a reasonable level of confidence to shoot for when making claims about whether the null hypothesis of no effect is likely to hold or not. Now there certainly are many problems associated with the hypothesis testing framework–some of them very serious–but if you really believe that “there’s no logical basis for using a P value from a single study to draw any conclusion,” your beef isn’t actually with p values, it’s with the very underpinnings of the scientific enterprise.

Anyway, the bottom line is Siegfried’s article is not so much bad as irresponsible. As an accessible description of some serious problems with common statistical practices, it’s actually quite good. But I guess the sense I got in reading the article was that at some point Siegfried became more interested in writing a contrarian piece about how scientists are falling down on the job than about how doing statistics well is just really hard for almost all of us (I certainly fail at it all the time!). And ironically, in the process of trying to explain just why “science fails to face the shortcomings of statistics”, Siegfried commits some of the very same errors he’s taking scientists to task for.

[UPDATE: Steve Novella says much the same thing here.]

[UPDATE 2: Andrew Gelman has a nice roundup of other comments on Siegfried’s article throughout the blogosphere.]

what the general factor of intelligence is and isn’t, or why intuitive unitarianism is a lousy guide to the neurobiology of higher cognitive ability

This post shamelessly plagiarizes liberally borrows ideas from a much longer, more detailed, and just generally better post by Cosma Shalizi. I’m not apologetic, since I’m a firm believer in the notion that good ideas should be repeated often and loudly. So I’m going to be often and loud here, though I’ll try to be (slightly) more succinct than Shalizi. Still, if you have the time to spare, you should read his longer and more mathematical take.

There’s a widely held view among intelligence researchers in particular, and psychologists more generally, that there’s a general factor of intelligence (often dubbed g) that accounts for a very large portion of the variance in a broad range of cognitive performance tasks. Which is to say, if you have a bunch of people do a bunch of different tasks, all of which we think tap different aspects of intellectual ability, and then you take all those scores and factor analyze them, you’ll almost invariably get a first factor that explains 50% or more of the variance in the zero-order scores. Or to put it differently, if you know a person’s relative standing on g, you can make a reasonable prediction about how that person will do on lots of different tasks–for example, digit symbol substitution, N-back, go/no-go, and so on and so forth. Virtually all tasks that we think reflect cognitive ability turn out, to varying extents, to reflect some underlying latent variable, and that latent variable is what we dub g.

In a trivial sense, no one really disputes that there’s such a thing as g. You can’t really dispute the existence of g, seeing as a general factor tends to fall out of virtually all factor analyses of cognitive tasks; it’s about as well-replicated a finding as you can get. To say that g exists, on the most basic reading, is simply to slap a name on the empirical fact that scores on different cognitive measures tend to intercorrelate positively to a considerable extent.

What’s not so clear is what the implications of g are for our understanding of how the human mind and brain works. If you take the presence of g at face value, all it really says is what we all pretty much already know: some people are smarter than others. People who do well in one intellectual domain will tend to do pretty well in others too, other things being equal. With the exception of some people who’ve tried to argue that there’s no such thing as general intelligence, but only “multiple intelligences” that totally fractionate across domains (not a compelling story, if you look at the evidence), it’s pretty clear that cognitive abilities tend to hang together pretty well.

The trouble really crops up when we try to say something interesting about the architecture of the human mind on the basis of the psychometric evidence for g. If someone tells you that there’s a single psychometric factor that explains at least 50% of the variance in a broad range of human cognitive abilities, it seems perfectly reasonable to suppose that that’s because there’s some unitary intelligence system in people’s heads, and that that system varies in capacity across individuals. In other words, the two intuitive models people have about intelligence seem to be that either (a) there’s some general cognitive system that corresponds to g, and supports a very large portion of the complex reasoning ability we call “intelligence” or (b) there are lots of different (and mostly unrelated) cognitive abilities, each of which contributes only to specific types of tasks and not others. Framed this way, it just seems obvious that the former view is the right one, and that the latter view has been discredited by the evidence.

The problem is that the psychometric evidence for g stems almost entirely from statistical procedures that aren’t really supposed to be use for causal inference. The primary weapon in the intelligence researcher’s toolbox has historically been principal components analysis (PCA) or exploratory factor analysis, which are really just data reduction techniques. PCA tells you how you can describe your data in a more compact way, but it doesn’t actually tell you what structure is in your data. A good analogy is the use of digital compression algorithms. If you take a directory full of .txt files and compress them into a single .zip file, you’ll almost certainly end up with a file that’s only a small fraction of the total size of the original texts. The reason this works is because certain patterns tend to repeat themselves over and over in .txt files, and a smart algorithm will store an abbreviated description of those patterns rather than the patterns themselves. Which, conceptually, is almost exactly what happens when you run a PCA on a dataset: you’re searching for consistent patterns in the way observations vary along multiple variables, and discarding any redundancy you come across in favor of a more compact description.

Now, in a very real sense, compression is impressive. It’s certainly nice to be able to email your friend a 140kb .zip of your 1200-page novel rather than a 2mb .doc. But note that you don’t actually learn much from the compression. It’s not like your friend can open up that 140k binary representation of your novel, read it, and spare herself the torture of the other 1860kb. If you want to understand what’s going on in a novel, you need to read the novel and think about the novel. And if you want to understand what’s going on in a set of correlations between different cognitive tasks, you need to carefully inspect those correlations and carefully think about those correlations. You can run a factor analysis if you like, and you might learn something, but you’re not going to get any deep insights into the “true” structure of the data. The “true” structure of the data is, by definition, what you started out with (give or take some error). When you run a PCA, you actually get a distorted (but simpler!) picture of the data.

To most people who use PCA, or other data reduction techniques, this isn’t a novel insight by any means. Most everyone who uses PCA knows that in an obvious sense you’re distorting the structure of the data when you reduce its dimensionality. But the use of data reduction is often defended by noting that there must be some reason why variables hang together in such a way that they can be reduced to a much smaller set of variables with relatively little loss of variance. In the context of intelligence, the intuition can be expressed as: if there wasn’t really a single factor underlying intelligence, why would we get such a strong first factor? After all, it didn’t have to turn out that way; we could have gotten lots of smaller factors that appear to reflect distinct types of ability, like verbal intelligence, spatial intelligence, perceptual speed, and so on. But it did turn out that way, so that tells us something important about the unitary nature of intelligence.

This is a strangely compelling argument, but it turns out to be only minimally true. What the presence of a strong first factor does tell you is that you have a lot of positively correlated variables in your data set. To be fair, that is informative. But it’s only minimally informative, because, assuming you eyeballed the correlation matrix in the original data, you already knew that.

What you don’t know, and can’t know, on the basis of a PCA, is what underlying causal structure actually generated the observed positive correlations between your variables. It’s certainly possible that there’s really only one central intelligence system that contributes the bulk of the variance to lots of different cognitive tasks. That’s the g model, and it’s entirely consistent with the empirical data. Unfortunately, it’s not the only one. To the contrary, there are an infinite number of possible causal models that would be consistent with any given factor structure derived from a PCA, including a structure dominated by a strong first factor. In fact, you can have a causal structure with as many variables as you like be consistent with g-like data. So long as the variables in your model all make contributions in the same direction to the observed variables, you will tend to end up with an excessively strong first factor. So you could in principle have 3,000 distinct systems in the human brain, all completely independent of one another, and all of which contribute relatively modestly to a bunch of different cognitive tasks. And you could still get a first factor that accounts for 50% or more of the variance. No g required.

If you doubt this is true, go read Cosma Shalizi’s post, where he not only walks you through a more detailed explanation of the mathematical necessity of this claim, but also illustrates the point using some very simple simulations. Basically, he builds a toy model in which 11 different tasks each draw on several hundred underlying cognitive tasks, which are turn drawn from a larger pool of 2,766 completely independent abilities. He then runs a PCA on the data and finds, lo and behold, a single factor that explains nearly 50% of the variance in scores. Using PCA, it turns out, you can get something huge from (almost) nothing.

Now, at this point a proponent of a unitary g might say, sure, it’s possible that there isn’t really a single cognitive system underlying variation in intelligence; but it’s not plausible, because it’s surely more parsimonious to posit a model with just one variable than a model with 2,766. But that’s only true if you think that our brains evolved in order to make life easier for psychometricians, which, last I checked, wasn’t the case. If you think even a little bit about what we know about the biological and genetic bases of human cognition, it starts to seem really unlikely that there really could be a single central intelligence system. For starters, the evidence just doesn’t support it. In the cognitive neuroscience literature, for example, biomarkers of intelligence abound, and they just don’t seem all that related. There’s a really nice paper in Nature Reviews Neuroscience this month by Deary, Penke, and Johnson that reviews a substantial portion of the literature of intelligence; the upshot is that intelligence has lots of different correlates. For example, people who score highly on intelligence tend to (a) have larger brains overall; (b) show regional differences in brain volume; (c) show differences in neural efficiency when performing cognitive tasks; (d) have greater white matter integrity; (e) have brains with more efficient network structures;  and so on.

These phenomena may not all be completely independent, but it’s hard to believe there’s any plausible story you could tell that renders them all part of some unitary intelligence system, or subject to unitary genetic influence. And really, why should they be part of a unitary system? Is there really any reason to think there has to be a single rate-limiting factor on performance? It’s surely perfectly plausible (I’d argue, much more plausible) to think that almost any complex cognitive task you use as an index of intelligence is going to draw on many, many different cognitive abilities. Take a trivial example: individual differences in visual acuity probably make a (very) small contribution to performance on many different cognitive tasks. If you can’t see the minute details of the stimuli as well as the next person, you might perform slightly worse on the task. So some variance in putatively “cognitive” task performance undoubtedly reflects abilities that most intelligence researchers wouldn’t really consider properly reflective of higher cognition at all. And yet, that variance has to go somewhere when you run a factor analysis. Most likely, it’ll go straight into that first factor, or g, since it’s variance that’s common to multiple tasks (i.e., someone with poorer eyesight may tend to do very slightly worse on any task that requires visual attention). In fact, any ability that makes unidirectional contributions to task performance, no matter how relevant or irrelevant to the conceptual definition of intelligence, will inflate the so-called g factor.

If this still seems counter-intuitive to you, here’s an analogy that might, to borrow Dan Dennett’s phrase, prime your intuition pump (it isn’t as dirty as it sounds). Imagine that instead of studying the relationship between different cognitive tasks, we decided to study the relation between performance at different sports. So we went out and rounded up 500 healthy young adults and had them engage in 16 different sports, including basketball, soccer, hockey, long-distance running, short-distance running, swimming, and so on. We then took performance scores for all 16 tasks and submitted them to a PCA. What do you think would happen? I’d be willing to bet good money that you’d get a strong first factor, just like with cognitive tasks. In other words, just like with g, you’d have one latent variable that seemed to explain the bulk of the variance in lots of different sports-related abilities. And just like g, it would have an easy and parsimonious interpretation: a general factor of athleticism!

Of course, in a trivial sense, you’d be right to call it that. I doubt anyone’s going to deny that some people just are more athletic than others. But if you then ask, “well, what’s the mechanism that underlies athleticism,” it’s suddenly much less plausible to think that there’s a single physiological variable or pathway that supports athleticism. In fact, it seems flatly absurd. You can easily think of dozens if not hundreds of factors that should contribute a small amount of the variance to performance on multiple sports. To name just a few: height, jumping ability, running speed, oxygen capacity, fine motor control, gross motor control, perceptual speed, response time, balance, and so on and so forth. And most of these are individually still relatively high-level abilities that break down further at the physiological level (e.g., “balance” is itself a complex trait that at minimum reflects contributions of the vestibular, visual, and cerebellar systems, and so on.). If you go down that road, it very quickly becomes obvious that you’re just not going to find a unitary mechanism that explains athletic ability. Because it doesn’t exist.

All of this isn’t to say that intelligence (or athleticism) isn’t “real”. Intelligence and athleticism are perfectly real; it makes complete sense, and is factually defensible, to talk about some people being smarter or more athletic than other people. But the point is that those judgments are based on superficial observations of behavior; knowing that people’s intelligence or athleticism may express itself in a (relatively) unitary fashion doesn’t tell you anything at all about the underlying causal mechanisms–how many of them there are, or how they interact.

As Cosma Shalizi notes, it also doesn’t tell you anything about heritability or malleability. The fact that we tend to think intelligence is highly heritable doesn’t provide any evidence in favor of a unitary underlying mechanism; it’s just as plausible to think that there are many, many individual abilities that contribute to complex cognitive behavior, all of which are also highly heritable individually. Similarly, there’s no reason to think our cognitive abilities would be any less or any more malleable depending on whether they reflect the operation of a single system or hundreds of variables. Regular physical exercise clearly improves people’s capacity to carry out all sorts of different activities, but that doesn’t mean you’re only training up a single physiological pathway when you exercise; a whole host of changes are taking place throughout your body.

So, assuming you buy the basic argument, where does that leave us? Depends. From a day-to-day standpoint, nothing changes. You can go on telling your friends that so-and-so is a terrific athlete but not the brightest crayon in the box, and your friends will go on understanding exactly what you meant. No one’s suggesting that intelligence isn’t stable and trait-like, just that, at the biological level, it isn’t really one stable trait.

The real impact of relaxing the view that g is a meaningful construct at the biological level, I think, will be in removing an artificial and overly restrictive constraint on researchers’ theorizing. The sense I get, having done some work on executive control, is that g is the 800-pound gorilla in the room: researchers interested in studying the neural bases of intelligence (or related constructs like executive or cognitive control) are always worrying about how their findings relate to g, and how to explain the fact that there might be dissociable neural correlates of different abilities (or even multiple independent contributions to fluid intelligence). To show you that I’m not making this concern up, and that it weighs heavily on many researchers, here’s a quote from the aforementioned and otherwise really excellent NRN paper by Deary et al reviewing recent findings on the neural bases of intelligence:

The neuroscience of intelligence is constrained by — and must explain — the following established facts about cognitive test performance: about half of the variance across varied cognitive tests is contained in general cognitive ability; much less variance is contained within broad domains of capability; there is some variance in specific abilities; and there are distinct ageing patterns for so-called fluid and crystallized aspects of cognitive ability.

The existence of g creates a complicated situation for neuroscience. The fact that g contributes substantial variance to all specific cognitive ability tests is generally thought to indicate that g contributes directly in some way to performance on those tests. That is, when domains of thinking skill (such as executive function and memory) or specific tasks (such as mental arithmetic and non-verbal reasoning on the Raven’s Progressive Matrices test) are studied, neuroscientists are observing brain activity related to g as well as the specific task activities. This undermines the ability to determine localized brain activities that are specific to the task at hand.

I hope I’ve convinced you by this point that the neuroscience of intelligence doesn’t have to explain why half of the variance is contained in general cognitive ability, because there’s no good evidence that there is such a thing as general cognitive ability (except in the descriptive psychometric sense, which carries no biological weight). Relaxing this artificial constraint would allow researchers to get on with the interesting and important business of identifying correlates (and potential causal determinants) of different cognitive abilities without having to worry about the relation of their finding to some Grand Theory of Intelligence. If you believe in g, you’re going to be at a complete loss to explain how researchers can continually identify new biological and genetic correlates of intelligence, and how the effect sizes could be so small (particularly at a genetic level, where no one’s identified a single polymorphism that accounts for more than a fraction of the observable variance in intelligence–the so called problem of “missing heritability”). But once you discard the fiction of g, you can take such findings in stride, and can set about the business of building integrative models that allow for and explicitly model the presence of multiple independent contributions to intelligence. And if studying the brain has taught us anything at all, it’s that the truth is inevitably more complicated than what we’d like to believe.

functional MRI and the many varieties of reliability

Craig Bennett and Mike Miller have a new paper on the reliability of fMRI. It’s a nice review that I think most people who work with fMRI will want to read. Bennett and Miller discuss a number of issues related to reliability, including why we should care about the reliability of fMRI, what factors influence reliability, how to obtain estimates of fMRI reliability, and what previous studies suggest about the reliability of fMRI. Their bottom line is that the reliability of fMRI often leaves something to be desired:

One thing is abundantly clear: fMRI is an effective research tool that has opened broad new horizons of investigation to scientists around the world. However, the results from fMRI research may be somewhat less reliable than many researchers implicitly believe. While it may be frustrating to know that fMRI results are not perfectly replicable, it is beneficial to take a longer-term view regarding the scientific impact of these studies. In neuroimaging, as in other scientific fields, errors will be made and some results will not replicate.

I think this is a wholly appropriate conclusion, and strongly recommend reading the entire article. Because there’s already a nice write-up of the paper over at Mind Hacks, I’ll content myself to adding a number of points to B&M’s discussion (I talk about some of these same issues in a chapter I wrote with Todd Braver).

First, even though I agree enthusiastically with the gist of B&M’s conclusion, it’s worth noting that, strictly speaking, there’s actually no such thing as “the reliability of fMRI”. Reliability isn’t a property of a technique or instrument, it’s a property of a specific measurement. Because every measurement is made under slightly different conditions, reliability will inevitably vary on a case-by-case basis. But since it’s not really practical (or even possible) to estimate reliability for every single analysis, researchers take necessary short-cuts. The standard in the psychometric literature is to establish reliability on a per-measure (not per-method!) basis, so long as conditions don’t vary too dramatically across samples. For example, once someone “validates” a given self-report measure, it’s generally taken for granted that that measure is “reliable”, and most people feel comfortable administering it to new samples without having to go to the trouble of estimating reliability themselves. That’s a perfectly reasonable approach, but the critical point is that it’s done on a relatively specific basis. Supposing you made up a new self-report measure of depression from a set of items you cobbled together yourself, you wouldn’t be entitled to conclude that your measure was reliable simply because some other self-report measure of depression had already been psychometrically validated. You’d be using an entirely new set of items, so you’d have to go to the trouble of validating your instrument anew.

By the same token, the reliability of any given fMRI measurement is going to fluctuate wildly depending on the task used, the timing of events, and many other factors. That’s not just because some estimates of reliability are better than others; it’s because there just isn’t a fact of the matter about what the “true” reliability of fMRI is. Rather, there are facts about how reliable fMRI is for specific types of tasks with specific acquisition parameters and preprocessing streams in specific scanners, and so on (which can then be summarized by talking about the general distribution of fMRI reliabilities). B&M are well aware of this point, and discuss it in some detail, but I think it’s worth emphasizing that when they say that “the results from fMRI research may be somewhat less reliable than many researchers implicitly believe,” what they mean isn’t that the “true” reliability of fMRI is likely to be around .5; rather, it’s that if you look at reliability estimates across a bunch of different studies and analyses, the estimated reliability is often low. But it’s not really possible to generalize from this overall estimate to any particular study; ultimately, if you want to know whether your data were measured reliably, you need to quantify that yourself. So the take-away message shouldn’t be that fMRI is an inherently unreliable method (and I really hope that isn’t how B&M’s findings get reported by the mainstream media should they get picked up), but rather, that there’s a very good chance that the reliability of fMRI in any given situation is not particularly high. It’s a subtle difference, but an important one.

Second, there’s a common misconception that reliability estimates impose an upper bound on the true detectable effect size. B&M make this point in their review, Vul et al made it in their “voodoo correlations”” paper, and in fact, I’ve made it myself before. But it’s actually not quite correct. It’s true that, for any given test, the true reliability of the variables involved limits the potential size of the true effect. But there are many different types of reliability, and most will generally only be appropriate and informative for a subset of statistical procedures. Virtually all types of reliability estimate will underestimate the true reliability in some cases and overestimate it in others. And in extreme cases, there may be close to zero relationship between the estimate and the truth.

To see this, take the following example, which focuses on internal consistency. Suppose you have two completely uncorrelated items, and you decide to administer them together as a single scale by simply summing up their scores. For example, let’s say you have an item assessing shoelace-tying ability, and another assessing how well people like the color blue, and you decide to create a shoelace-tying-and-blue-preferring measure. Now, this measure is clearly nonsensical, in that it’s unlikely to predict anything you’d ever care about. More important for our purposes, its internal consistency would be zero, because its items are (by hypothesis) uncorrelated, so it’s not measuring anything coherent. But that doesn’t mean the measure is unreliable! So long as the constituent items are each individually measured reliably, the true reliability of the total score could potentially be quite high, and even perfect. In other words, if I can measure your shoelace-tying ability and your blueness-liking with perfect reliability, then by definition, I can measure any linear combination of those two things with perfect reliability as well. The result wouldn’t mean anything, and the measure would have no validity, but from a reliability standpoint, it’d be impeccable. This problem of underestimating reliability when items are heterogeneous has been discussed in the psychometric literature for at least 70 years, and yet you still very commonly see people do questionable things like “correcting for attenuation” based on dubious internal consistency estimates.

In their review, B&M mostly focus on test-retest reliability rather than internal consistency, but the same general point applies. Test-retest reliability is the degree to which people’s scores on some variable are consistent across multiple testing occasions. The intuition is that, if the rank-ordering of scores varies substantially across occasions (e.g., if the people who show the highest activation of visual cortex at Time 1 aren’t the same ones who show the highest activation at Time 2), the measurement must not have been reliable, so you can’t trust any effects that are larger than the estimated test-retest reliability coefficient. The problem with this intuition is that there can be any number of systematic yet session-specific influences on a person’s score on some variable (e.g., activation level). For example, let’s say you’re doing a study looking at the relation between performance on a difficult working memory task and frontoparietal activation during the same task. Suppose you do the exact same experiment with the same subjects on two separate occasions three weeks apart, and it turns out that the correlation between DLPFC activation across the two occasions is only .3. A simplistic view would be that this means that the reliability of DLPFC activation is only .3, so you couldn’t possibly detect any correlations between performance level and activation greater than .3 in DLPFC. But that’s simply not true. It could, for example, be that the DLPFC response during WM performance is perfectly reliable, but is heavily dependent on session-specific factors such as baseline fatigue levels, motivation, and so on. In other words, there might be a very strong and perfectly “real” correlation between WM performance and DLPFC activation on each of the two testing occasions, even though there’s very little consistency across the two occasions. Test-retest reliability estimates only tell you how much of the signal is reliably due to temporally stable variables, and not how much of the signal is reliable, period.

The general point is that you can’t just report any estimate of reliability that you like (or that’s easy to calculate) and assume that tells you anything meaningful about the likelihood of your analyses succeeding. You have to think hard about exactly what kind of reliability you care about, and then come up with an estimate to match that. There’s a reasonable argument to be made that most of the estimates of fMRI reliability reported to date are actually not all that relevant to many people’s analyses, because the majority of reliability analyses have focused on test-retest reliability, which is only an appropriate way to estimate reliability if you’re trying to relate fMRI activation to stable trait measures (e.g., personality or cognitive ability). If you’re interested in relating in-scanner task performance or state-dependent variables (e.g., mood) to brain activation (arguably the more common approach), or if you’re conducting within-subject analyses that focus on comparisons between conditions, using test-retest reliability isn’t particularly informative, and you really need to focus on other types of reliability (or reproducibility).

Third, and related to the above point, between-subject and within-subject reliability are often in statistical tension with one another. B&M don’t talk about this, as far as I can tell, but it’s an important point to remember when designing studies and/or conducting analyses. Essentially, the issue is that what counts as error depends on what effects you’re interested in. If you’re interested in individual differences, it’s within-subject variance that counts as error, so you want to minimize that. Conversely, if you’re interested in within-subject effects (the norm in fMRI), you want to minimize between-subject variance. But you generally can’t do both of these at the same time. If you use a very “strong” experimental manipulation (i.e., a task that produces a very large difference between conditions for virtually all subjects), you’re going to reduce the variability between individuals, and you may very well end up with very low test-retest reliability estimates. And that would actually be a good thing! Conversely, if you use a “weak” experimental manipulation, you might get no mean effect at all, because there’ll be much more variability between individuals. There’s no right or wrong here; the trick is to pick a design that matches the focus of your study. In the context of reliability, the essential point is that if all you’re interested in is the contrast between high and low working memory load, it shouldn’t necessarily bother you if someone tells you that the test-retest reliability of induced activation in your study is close to zero. Conversely, if you care about individual differences, it shouldn’t worry you if activations aren’t reproducible across studies at the group level. In some ways, those are actual the ideal situations for each of those two types of studies.

Lastly, B&M raise a question as to what level of reliability we should consider “acceptable” for fMRI research:

There is no consensus value regarding what constitutes an acceptable level of reliability in fMRI. Is an ICC value of 0.50 enough? Should studies be required to achieve an ICC of 0.70? All of the studies in the review simply reported what the reliability values were. Few studies proposed any kind of criteria to be considered a “˜reliable’ result. Cicchetti and Sparrow did propose some qualitative descriptions of data based on the ICC-derived reliability of results (1981). They proposed that results with an ICC above 0.75 be considered “˜excellent’, results between 0.59 and 0.75 be considered “˜good’, results between .40 and .58 be considered “˜fair’, and results lower than 0.40 be considered “˜poor’. More specifically to neuroimaging, Eaton et al. (2008) used a threshold of ICC > 0.4 as the mask value for their study while Aron et al. (2006) used an ICC cutoff of ICC > 0.5 as the mask value.

On this point, I don’t really see any reason to depart from psychometric convention just because we’re using fMRI rather than some other technique. Conventionally, reliability estimates of around .8 (or maybe .7, if you’re feeling generous) are considered adequate. Any lower and you start to run into problems, because effect sizes will shrivel up. So I think we should be striving to attain the same levels of reliability with fMRI as with any other measure. If it turns out that that’s not possible, we’ll have to live with that, but I don’t think the solution is to conclude that reliability estimates on the order of .5 are ok “for fMRI” (I’m not saying that’s what B&M say, just that that’s what we should be careful not to conclude). Rather, we should just accept that the odds of detecting certain kinds of effects with fMRI are probably going to be lower than with other techniques. And maybe we should minimize the use of fMRI for those types of analyses where reliability is generally not so good (e.g., using brain activation to predict trait variables over long intervals).

I hasten to point out that none of this should be taken as a criticism of B&M’s paper; I think all of these points complement B&M’s discussion, and don’t detract in any way from its overall importance. Reliability is a big topic, and there’s no way Bennett and Miller could say everything there is to be said about it in one paper. I think they’ve done the field of cognitive neuroscience an important service by raising awareness and providing an accessible overview of some of the issues surrounding reliability, and it’s certainly a paper that’s going on my “essential readings in fMRI methods” list.

ResearchBlogging.org
Bennett, C. M., & Miller, M. B. (2010). How reliable are the results from functional magnetic resonance imaging? Annals of the New York Academy of Sciences

the OKCupid guide to dating older women

Continuing along on their guided tour of Data I Wish I Had Access To, the OKCupid folks have posted another set of interesting figures on their blog. This time, they make the case for dating older women, suggesting that men might get more bang for their buck (in a literal sense, I suppose) by trying to contact women their age or older, rather than trying to hit on the young ‘uns. Men, it turns out, are creepy. Here’s how creepy:

Actually, that’s not so creepy. All it says is that men say they prefer to date younger women. That’s not going to shock anyone. This one is creepier:

The reason it’s creepy is that it basically says that, irrespective of what age ranges men say they find acceptable in a potential match, they’re actually all indiscriminately messaging 18-year old women. So basically, if you’re a woman on OKCupid who’s searching for that one special, non-creepy guy, be warned: they don’t exist. They’re pretty much all going to be eying 18-year olds for the rest of their lives. (To be fair, women also show a tendency to contact men below their lowest reported acceptable age. But it’s a much weaker effect; 40-year old women only occasionally try to hit on 24-year old guys, and tend to stay the hell away from the not-yet-of-drinking-age male population.)

Anyway, using this type of data, the OKCupid folks then generate this figure:

…which also will probably surprise no one, as it basically says women are most desirable when they’re young, and men when they’re (somewhat) older. But what the OKCupid folks then suggest is that it would be to men’s great advantage to broaden their horizons, because older women (which, in their range-restricted population, basically means anything over 30) self-report being much more interested in having sex more often, having casual sex, and using protection. I won’t bother hotlinking to all of those images, but here’s where they’re ultimately going with this:

I’m not going to comment on the appropriateness of trying to nudge one’s male userbase in the direction of more readily available casual sex (though I suspect they don’t need much nudging anyway). What I do wonder is to what extent these results reflect selection effects rather than a genuine age difference. The OKCupid folks suggest that women’s sexual interest increases as they age, which seems plausible given the conventional wisdom that women peak sexually in their 30s. But the effects in this case look pretty huge (unless the color scheme is misleading, which it might be; you’ll have to check out the post for the neat interactive flash animations), and it seems pretty plausible that much of the age effect could be driven by selection bias. Women with a more monogamous orientation are probably much more likely to be in committed, stable relationships by the time they turn 30 or 35, and probably aren’t scanning OKCupid for potential mates. Women who are in their 30s and 40s and still using online dating services are probably those who weren’t as interested in monogamous relationships to begin with. (Of course, the same is probably true of older men. Except that since men of all ages appear to be pretty interested in casual sex, there’s unlikely to be an obvious age differential.)

The other thing I’m not clear on is whether these analyses control for the fact that the userbase is heavily skewed toward younger users:

The people behind OKCupid are all mathematicians by training, so I’d be surprised if they hadn’t taken the underlying age distribution into consideration. But they don’t say anything about it in their post. The worry is that, if the base rate of different age groups isn’t taken into consideration, the heat map displayed above could be quite misleading. Given that there are many, many more 25-year old women on OKCupid than 35-year old women, failing to normalize properly would almost invariably make it look like there’s a heavy skew for men to message relatively younger women, irrespective of the male sender’s age. By the same token, it’s not clear that it’d be good advice to tell men to seek out older women, given that there are many fewer older women in the pool to begin with. As a thought experiment, suppose that the entire OKCupid male population suddenly started messaging women 5 years older than them, and entirely ignored their usual younger targets. The hit rate wouldn’t go up; it would probably actually fall precipitously, since there wouldn’t be enough older women to keep all the younger men entertained (at least, I certainly hope there wouldn’t). No doubt there’s a stable equilibrium point somewhere, where men and women are each targeting exactly the right age range to maximize their respective chances. I’m just not sure that it’s in OKCupid’s proposed “zone of greatness” for the men.

It’s also a bit surprising that OKCupid didn’t break down the response rate to people of the opposite gender as a function of the sender and receiver’s age. They’ve done this in the past, and it seems like the most direct way of testing whether men are more likely to get lucky by messaging older or younger women. Without knowing whether older women are actually responding to younger men’s overtures, it’s kind of hard to say what it all means. Except that I’d still kill to have their data.

the parable of zoltan and his twelve sheep, or why a little skepticism goes a long way

What follows is a fictional piece about sheep and statistics. I wrote it about two years ago, intending it to serve as a preface to an article on the dangers of inadvertent data fudging. But then I decided that no journal editor in his or her right mind would accept an article that started out talking about thinking sheep. And anyway, the rest of the article wasn’t very good. So instead, I post this parable here for your ovine amusement. There’s a moral to the story, but I’m too lazy to write about it at the moment.

A shepherd named Zoltan lived in a small village in the foothills of the Carpathian Mountains. He tended to a flock of twelve sheep: Soffia, Krystyna, Anastasia, Orsolya, Marianna, Zigana, Julinka, Rozalia, Zsa Zsa, Franciska, Erzsebet, and Agi. Zoltan was a keen observer of animal nature, and would often point out the idiosyncracies of his sheep’s behavior to other shepherds whenever they got together.

“Anastasia and Orsolya are BFFs. Whatever one does, the other one does too. If Anastasia starts licking her face, Orsolya will too; if Orsolya starts bleating, Anastasia will start harmonizing along with her.“

“Julinka has a limp in her left leg that makes her ornery. She doesn’t want your pity, only your delicious clovers.“

“Agi is stubborn but logical. You know that old saying, spare the rod and spoil the sheep? Well, it doesn’t work for Agi. You need calculus and rhetoric with Agi.“

Zoltan’s colleagues were so impressed by these insights that they began to encourage him to record his observations for posterity.

“Just think, Zoltan,“ young Gergely once confided. “If something bad happened to you, the world would lose all of your knowledge. You should write a book about sheep and give it to the rest of us. I hear you only need to know six or seven related things to publish a book.“

On such occasions, Zoltan would hem and haw solemnly, mumbling that he didn’t know enough to write a book, and that anyway, nothing he said was really very important. It was false modestly of course; in reality, he was deeply flattered, and very much concerned that his vast body of sheep knowledge would disappear along with him one day. So one day, Zoltan packed up his knapsack, asked Gergely to look after his sheep for the day, and went off to consult with the wise old woman who lived in the next village.

The old woman listened to Zoltan’s story with a good deal of interest, nodding sagely at all the right moments. When Zoltan was done, the old woman mulled her thoughts over for a while.

“If you want to be taken seriously, you must publish your findings in a peer-reviewed journal,” she said finally.

“What’s Pier Evew?” asked Zoltan.

“One moment,” said the old woman, disappearing into her bedroom. She returned clutching a dusty magazine. “Here,” she said, handing the magazine to Zoltan. “This is peer review.”

That night, after his sheep had gone to bed, Zoltan stayed up late poring over Vol. IV, Issue 5 of Domesticated Animal Behavior Quarterly. Since he couldn’t understand the figures in the magazine, he read it purely for the articles. By the time he put the magazine down and leaned over to turn off the light, the first glimmerings of an empirical research program had begun to dance around in his head. Just like fireflies, he thought. No, wait, those really were fireflies. He swatted them away.

“I like this“¦ science,” he mumbled to himself as he fell asleep.

In the morning, Zoltan went down to the local library to find a book or two about science. He checked out a volume entitled Principia Scientifica Buccolica—a masterful derivation from first principles of all of the most common research methods, with special applications to animal behavior. By lunchtime, Zoltan had covered t-tests, and by bedtime, he had mastered Mordenkainen’s correction for inestimable herds.

In the morning, Zoltan made his first real scientific decision.

“Today I’ll collect some pilot data,” he thought to himself, “and tomorrow I’ll apply for an R01.”

His first set of studies tested the provocative hypothesis that sheep communicate with one another by moving their ears back and forth in Morse code. Study 1 tested the idea observationally. Zoltan and two other raters (his younger cousins), both blind to the hypothesis, studied sheep in pairs, coding one sheep’s ear movements and the other sheep’s behavioral responses. Studies 2 through 4 manipulated the sheep’s behavior experimentally. In Study 2, Zoltan taped the sheep’s ears to their head; in Study 3, he covered their eyes with opaque goggles so that they couldn’t see each other’s ears moving. In Study 4, he split the twelve sheep into three groups of four in order to determine whether smaller groups might promote increased sociability.

That night, Zoltan minded the data. “It’s a lot like minding sheep,“ Zoltan explained to his cousin Griga the next day. “You need to always be vigilant, so that a significant result doesn’t get away from you.“

Zoltan had been vigilant, and the first 4 studies produced a number of significant results. In Study 1, Zoltan found that sheep appeared to coordinate ear twitches: if one sheep twitched an ear several times in a row, it was a safe bet that other sheep would start to do the same shortly thereafter (p < .01). There was, however, no coordination of licking, headbutting, stamping, or bleating behaviors, no matter how you sliced and diced it. “It’s a highly selective effect,“ Zoltan concluded happily. After all, when you thought about it, it made sense. If you were going to pick just one channel for sheep to communicate through, ear twitching was surely a good one. One could make a very good evolutionary argument that more obvious methods of communication (e.g., bleating loudly) would have been detected by humans long ago, and that would be no good at all for the sheep.

Studies 2 and 3 further supported Zoltan’s story. Study 2 demonstrated that when you taped sheep’s ears to their heads, they ceased to communicate entirely. You could put Rozalia and Erzsebet in adjacent enclosures and show Rozalia the Jack of Spades for three or four minutes at a time, and when you went to test Erzsebet, she still wouldn’t know the Jack of Spades from the Three of Diamonds. It was as if the sheep were blind! Except they weren’t blind, they were dumb. Zoltan knew; he had made them that way by taping their ears to their heads.

In Study 3, Zoltan found that when the sheep’s eyes were covered, they no longer coordinated ear twitching. Instead, they now coordinated their bleating—but only if you excluded bleats that were produced when the sheep’s heads were oriented downwards. “Fantastic,“ he thought. “When you cover their eyes, they can’t see each other’s ears any more. So they use a vocal channel. This, again, makes good adaptive sense: communication is too important to eliminate entirely just because your eyes happen to be covered. Much better to incur a small risk of being detected and make yourself known in other, less subtle, ways.“

But the real clincher was Study 4, which confirmed that ear twitching occurred at a higher rate in smaller groups than larger groups, and was particularly common in dyads of well-adjusted sheep (like Anastasia and Orsolya, and definitely not like Zsa Zsa and Marianna).

“Sheep are like everyday people,“ Zoltan told his sister on the phone. “They won’t say anything to your face in public, but get them one-on-one, and they won’t stop gossiping about each other.“

It was a compelling story, Zoltan conceded to himself. The only problem was the F test. The difference in twitch rates as a function of group size wasn’t quite statistically significant. Instead, it hovered around p = .07, which the textbooks told Zoltan meant that he was almost right. Almost right was the same thing as potentially wrong, which wasn’t good enough. So the next morning, Zoltan asked Gergely to lend him four sheep so he could increase his sample size.

“Absolutely not,“ said Gergely. “I don’t want your sheep filling my sheep’s heads with all of your crazy new ideas.“

“Look,“ said Zoltan. “If you lend me four sheep, I’ll let you drive my Cadillac down to the village on weekends after I get famous.“

“Deal,“ said Gergely.

So Zoltan borrowed the sheep. But it turned out that four sheep weren’t quite enough; after adding Gergely’s sheep to the sample, the effect only went from p < .07 to p < .06. So Zoltan cut a deal with his other neighbor, Yuri: four of Yuri’s sheep for two days, in return for three days with Zoltan’s new Lexus (once he bought it). That did the trick. Once Zoltan repeated the experiment with Yuri’s sheep, the p-value for Study 2 now came to .046, which the textbooks assured Zoltan meant he was going to be famous.

Data in hand, Zoltan spent the next two weeks writing up his very first journal article. He titled it “Baa baa baa, or not: Sheep communicate via non-verbal channels“—a decidedly modest title for the first empirical work to demonstrate that sheep are capable of sophisticated propositional thought. The article was published to widespread media attention and scientific acclaim, and Zoltan went on to have a productive few years in animal behavioral research, studying topics as interesting and varied as giraffe calisthenics and displays of affection in the common leech.

Much later, it turned out that no one was able to directly replicate his original findings with sheep (though some other researchers did manage to come up with conceptual replications). But that didn’t really matter to Zoltan, because by then he’d decided science was too demanding a career anyway; it was way more fun to lay under trees counting his sheep. Counting sheep, and occasionally, on Saturdays, driving down to the village in his new Lexus,  just to impress all the young cowgirls.

got R? get social science for R!

Drew Conway has a great list of 10 must-have R packages for social scientists. If you’re a social scientist (or really, any kind of scientist) who doesn’t use R, now is a great time to dive in and learn; there are tons of tutorials and guides out there (my favorite is Quick-R, which is incredibly useful incredibly often), and packages are available for just about any application you can think of. Best of all, R is completely free, and is available for just about every platform. Admittedly, there’s a fairly steep learning curve if you’re used to GUI-based packages like SPSS (R’s syntax can be pretty idiosyncratic), but it’s totally worth the time investment, and once you’re comfortable with R you’ll never look back.

Anyway, Drew’s list contains a number of packages I’ve found invaluable in my work, as well as several packages I haven’t used before and am pretty eager to try. I don’t have much to add to his excellent summaries, but I’ll gladly second the inclusion of ggplot2 (the easiest way in the world to make beautiful graphs?) and plyr and sqldf (great for sanitizing, organizing, and manipulating large data sets, which are often a source of frustration in R). Most of the other packages I haven’t had any reason to use personally, though a few seem really cool, and worth finding an excuse to play around with (e.g., Statnet and igraph).

Since Drew’s list focuses on packages useful to social scientists in general, I thought I’d mention a couple of others that I’ve found particularly useful for psychological applications. The most obvious one is William Revelle‘s awesome psych package, which contains tons of useful functions for descriptive statistics, data reduction, simulation, and psychometrics. It’s saved me me tons of time validating and scoring personality measures, though it probably isn’t quite as useful if you don’t deal with individual difference measures regularly. Other packages I’ve found useful are sem for structural equation modeling (which interfaces nicely with GraphViz to easily produce clean-looking path diagrams), genalg for genetic algorithms, MASS (mostly for sampling from multivariate distributions), reshape (similar functionality to plyr), and car, which contains a bunch of useful regression-related functions (e.g., for my dissertation, I needed to run SPSS-like repeated measures ANOVAs in R, which turns out to be a more difficult proposition than you’d imagine, but was handled by the Anova function in car). I’m sure there are others I’m forgetting, but those are the ones that I’ve relied on most heavily in recent work. No doubt there are tons of other packages out there that are handly for common psychology applications, so if there are any you use regularly, I’d love to hear about them in the comments!

specificity statistics for ROI analyses: a simple proposal

The brain is a big place. In the context of fMRI analysis, what that bigness means is that a typical 3D image of the brain might contain anywhere from 50,000 – 200,000 distinct voxels (3D pixels). Any of those voxels could theoretically show meaningful activation in relation to some contrast of interest, so the only way to be sure that you haven’t overlooked potentially interesting activations is to literally test every voxel (or, given some parcellation algorithm, every region).

Unfortunately, the problem that approach raises–which I’ve discussed in more detail here–is the familiar one of multiple comparisons: If you’re going to test 100,000 locations, it’s not really fair to test each one at the conventional level of p < .05, because on average, you’ll get about 5,000 statistically significant results just by chance that way. So you need to do something to correct for the fact that you’re running thousands of tests. The most common approach is to simply make the threshold for significance more conservative–for example, by testing at p < .0001 instead of p < .05, or by using some combination of intensity and cluster extent thresholds (e.g., you look for 20 contiguous voxels that are all significant at, say, p < .001) that’s supposed to guarantee a cluster-wise error rate of .05.

There is, however, a natural tension between false positives and false negatives: When you make your analysis more conservative, you let fewer false positives through the filter, but you also keep more of the true positives out. A lot of fMRI analysis really just boils down to walking a very thin line between running overconservative analyses that can’t detect anything but the most monstrous effects, and running overly liberal analyses that lack any real ability to distinguish meaningful signals from noise. One very common approach that fMRI researchers have adopted in an effort to optimize this balance is to use complementary hypothesis-driven and whole-brain analyses. The idea is that you’re basically carving the brain up into two separate search spaces: One small space for which you have a priori hypotheses that can be tested using a small number of statistical comparisons, and one much larger space (containing everything but the a priori space) where you continue to use a much more conservative threshold.

For example, if I believe that there’s a very specific chunk of right inferotemporal cortex that’s specialized for detecting clown faces, I can focus my hypothesis-testing on that particular region, without having to pretend that all voxels are created equal. So I delineate the boundaries of a CRC (Clown Representation Cortex) region-of-interest (ROI) based on some prior criteria (e.g., anatomy, or CRC activation in previous studies), and then I can run a single test at p < .05 to test my hypothesis, no correction needed. But to ensure that I don’t miss out on potentially important clown-related activation elsewhere in the brain, I also go ahead and run an additional whole-brain analysis that’s fully corrected for multiple comparisons. By coupling these two analyses, I hopefully get the best of both worlds. That is, I combine one approach (the ROI analysis) that maximizes power to test a priori hypotheses at the cost of an inability to detect effects in unexpected places with another approach (the whole-brain analysis) that has a much more limited capacity to detect effects in both expected and unexpected locations.

This two-pronged strategy is generally a pretty successful one, and I’d go so far as to say that a very large minority, if not an outright majority, of fMRI studies currently use it. Used wisely, I think it’s really an invaluable strategy. There is, however, one fairly serious and largely unappreciated problem associated with the incautious application of this approach. It has to do with claims about the specificity of activation that often tend to accompany studies that use a complementary ROI/whole-brain strategy. Specifically, a pretty common pattern is for researchers to (a) confirm their theoretical predictions by successfully detecting activation in one or more a priori ROIs; (b) identify few if any whole-brain activations; and consequently, (c) conclude that not only were the theoretical predictions confirmed, but that the hypothesized effects in the a priori ROIs were spatially selective, because a complementary whole-brain analysis didn’t turn up much (if anything). Or, to put it in less formal terms, not only were we right, we were really right! There isn’t any other part of the brain that shows the effect we hypothesized we’d see in our a priori ROI!

The problem with this type of inference is that there’s usually a massive discrepancy in the level of power available to detect effects in a priori ROIs versus the rest of the brain. If you search at p < .05 within some predetermined space, but at only p < .0001 everywhere else, you’re naturally going to detect results at a much lower rate everywhere else. But that’s not necessarily because there wasn’t just as much to look at everywhere else; it could just be because you didn’t look very carefully. By way of analogy, if you’re out picking berries in the forest, and you decide to spend half your time on just one bush that (from a distance) seemed particularly berry-full, and the other half of your time divided between the other 40 bushes in the area, you’re not really entitled to conclude that you picked the best bush all along simply because you came away with a relatively full basket. Had you done a better job checking out the other bushes, you might well have found some that were even better, and then you’d have come away carrying two baskets full of delicious, sweet, sweet berries.

Now, in an ideal world, we’d solve this problem by simply going around and carefully inspecting all the berry bushes, until we were berry, berry sure really convinced that we’d found all of the best bushes. Unfortunately, we can’t do that, because we’re out here collecting berries on our lunch break, and the boss isn’t paying us to dick around in the woods. Or, to return to fMRI World, we simply can’t carefully inspect every single voxel (say, by testing it at p < .05), because then we’re right back in mega-false-positive-land, which we’ve already established as a totally boring place we want to avoid at all costs.

Since an optimal solution isn’t likely, the next best thing is to figure out what we can do to guard against careless overinterpretation. Here I think there’s actually a very simple, and relatively elegant, solution. What I’ve suggested when I’ve given recent talks on this topic is that we mandate (or at least, encourage) the use of what you could call a specificity statistic (SS). The SS is a very simple measure of how specific a given ROI-level finding is; it’s just the proportion of voxels that are statistically significant when tested at the same level as the ROI-level effects. In most cases, that’s going to be p < .05, so the SS will usually just be the proportion of all voxels anywhere in the brain that are activated at p < .05.

To see why this is useful, consider what could no longer happen: Researchers would no longer be able to (inadvertently) capitalize on the fact that the one or two regions they happened to define as a priori ROIs turned up significant effects when no other regions did in a whole-brain analysis. Suppose that someone reports a finding that negative emotion activates the amygdala in an ROI analysis, but doesn’t activate any other region in a whole-brain analysis. (While I’m pulling this particular example out of a hat here, I feel pretty confident that if you went and did a thorough literature review, you’d find at least three or four studies that have made this exact claim.) This is a case where the SS would come in really handy. Because if the SS is, say, 26% (i.e., about a quarter of all voxels in the brain are active at p < .05, even if none survive full correction for multiple comparisons), you would want to draw a very different conclusion than if it was just 4%. If fully a quarter of the brain were to show greater activation for a negative-minus-neutral emotion contrast, you wouldn’t want to conclude that the amygdala was critically involved in negative emotion; a better interpretation would be that the researchers in question just happened to define an a priori region that fell within the right quarter of the brain. Perhaps all that’s happening is that negative emotion elicits a general increase in attention, and much of the brain (including, but by no means limited to, the amygdala) tends to increase activation correspondingly. So as a reviewer and reader, you’d want to know how specific the reported amygdala activation really is*. But in the vast majority of papers, you currently have no way of telling (and the researchers probably don’t even know the answer themselves!).

The principal beauty of this statistic lies in its simplicity: It’s easy to understand, easy to calculate, and easy to report. Ideally, researchers would report the SS any time ROI analyses are involved, and would do it for every reported contrast. But at minimum, I think we should all encourage each other (and ourselves) to report such a statistic any time we’re making a specificity claim about ROI-based results. In other words,if you want to argue that a particular cognitive function is relatively localized to the ROI(s) you happened to select, you should be required to show that there aren’t that many other voxels (or regions) that show the same effect when tested at the liberal threshold you used for the ROI analysis. There shouldn’t be an excuse for not doing this; it’s a very easy procedure for researchers to implement, and an even easier one for reviewers to demand.

* An alternative measure of specificity would be to report the percentile ranking of all of the voxels within the ROI mask relative to all other individual voxels. In the above example, you’d assign very different interpretations depending on whether the amygdala was in the 32nd or 87th percentile of all voxels, when ordered according to the strength of the effect for the negative – neutral contrast.

Ioannidis on effect size inflation, with guest appearance by Bozo the Clown

Andrew Gelman posted a link on his blog today to a paper by John Ioannidis I hadn’t seen before. In many respects, it’s basically the same paper I wrote earlier this year as a commentary on the Vul et al “voodoo correlations” paper (the commentary was itself based largely on an earlier chapter I wrote with my PhD advisor, Todd Braver). Well, except that the Ioannidis paper came out a year earlier than mine, and is also much better in just about every respect (more on this below).

What really surprises me is that I never came across Ioannidis’ paper when I was doing a lit search for my commentary. The basic point I made in the commentary–which can be summarized as the observation that low power coupled with selection bias almost invariably inflates significant effect sizes–is a pretty straightforward statistical point, so I figured that many people, and probably most statisticians, were well aware of it. But no amount of Google Scholar-ing helped me find an authoritative article that made the same point succinctly; I just kept coming across articles that made the point tangentially, in an off-hand “but of course we all know we shouldn’t trust these effect sizes, because…” kind of way. So I chalked it down as one of those statistical factoids (of which there are surprisingly many) that live in the unhappy land of too-obvious-for-statisticians-to-write-an-article-about-but-not-obvious-enough-for-most-psychologists-to-know-about. And so I just went ahead and wrote the commentary in a non-technical way that I hoped would get the point across intuitively.

Anyway, after the commentary was accepted, I sent a copy to Andrew Gelman, who had written several posts about the Vul et al controversy. He promptly send me back a link to this paper of his, which basically makes the same point about sampling error, but with much more detail and much better examples than I did. His paper also cites an earlier article in American Scientist by Wainer, which I also recommend, and again expresses very similar ideas. So then I felt a bit like a fool for not stumbling across either Gelman’s paper or Wainer’s earlier. And now that I’ve read Ioannidis’ paper, I feel even dumber, seeing as I could have saved myself a lot of trouble by writing two or three paragraphs and then essentially pointing to Ioannidis’ work. Oh well.

That all said, it wasn’t a complete loss; I still think the basic point is important enough that it’s worth repeating loudly and often, no matter how many times it’s been said before. And I’m skeptical that many fMRI researchers would have appreciated the point otherwise, given that none of the papers I’ve mentioned were published in venues fMRI researchers are likely to read regularly (which is presumably part of the reason I never came across them!). Of course, I don’t think that many people who do fMRI research actually bothered to read my commentary, so it’s questionable whether it had much impact anyway.

At any rate, the Ioannidis paper makes a number of points that my paper didn’t, so I figured I’d talk about them a bit. I’ll start by revisiting what I said in my commentary, and then I’ll tell you why you should read Ioannidis’ paper instead of mine.

The basic intuition can be captured as follows. Suppose you’re interested in the following question: Do clowns suffer depression at a higher rate than us non-comical folk do? You might think this is a contrived (to put it delicately) question, but I can assure you it has all sorts of important real-world implications. For instance, you wouldn’t be so quick to book a clown for your child’s next birthday party if you knew that The Great Mancini was going to be out in the parking lot half an hour later drinking cheap gin out of a top hat. If that example makes you feel guilty, congratulations: you’ve just discovered the translational value of basic science.

Anyway, back to the question, and how we’re going to answer it. You can’t just throw a bunch of clowns and non-clowns in a room and give them a depression measure. There’s nothing comical about that. What you need to do, if you’re rigorous about it, is give them multiple measures of depression, because we all know how finicky individual questionnaires can be. So the clowns and non-clowns each get to fill out the Beck Depression Inventory (BDI), the Center for Epidemiologic Studies Depression Scale, the Depression Adjective Checklist, the Zung Self-Rating Depression Scale (ZSRDS), and, let’s say, six other measures. Ten measures in all. And let’s say we have 20 individuals in each group, because that’s all I personally a cash-strapped but enthusiastic investigator can afford. After collecting the data, we score the questionnaires and run a bunch of t-tests to determine whether clowns and non-clowns have different levels of depression. Being scrupulous researchers who care a lot about multiple comparisons correction, we decide to divide our critical p-value by 10 (the dreaded Bonferroni correction, for 10 tests in this case) and test at p < .005. That’s a conservative analysis, of course; but better safe than sorry!

So we run our tests and get what look like mixed results. Meaning, we get statistically significant positive correlations between clown-dom status and depression for 2 measures–the BDI and Zung inventories–but not for the other 8 measures. So that’s admittedly not great; it would have been better if all 10 had come out right. Still, it at least partially supports our hypothesis: Clowns are fucking miserable! And because we’re already thinking ahead to how we’re going to present these results when they (inevitably) get published in Psychological Science, we go ahead and compute the effect sizes for the two significant correlations, because, after all, it’s important to know not only that there is a “real” effect, but also how big that effect is. When we do that, it turns out that the point-biserial correlation is huge! It’s .75 for the BDI and .68 for the ZSRDS. In other words, about half of the variance in clowndom can be explained by depression levels. And of course, because we’re well aware that correlation does not imply causation, we get to interpret the correlation both ways! So we quickly issue a press release claiming that we’ve discovered that it’s possible to conclusively diagnose depression just by knowing whether or not someone’s a clown! (We’re not going to worry about silly little things like base rates in a press release.)

Now, this may all seem great. And it’s probably not an unrealistic depiction of how much of psychology works (well, minus the colorful scarves, big hair, and face paint). That is, very often people report interesting findings that were selectively reported from amongst a larger pool of potential findings on the basis of the fact that the former but not the latter surpassed some predetermined criterion for statistical significance. For example, in our hypothetical in press clown paper, we don’t bother to report results for the correlation between clownhood and the Center for Epidemiologic Studies Depression Scale (r = .12, p > .1). Why should we? It’d be silly to report a whole pile of additional correlations only to turn around and say “null effect, null effect, null effect, null effect, null effect, null effect, null effect, and null effect” (see how boring it was to read that?). Nobody cares about variables that don’t predict other variables; we care about variables that do predict other variables. And we’re not really doing anything wrong, we think; it’s not like the act of selective reporting is inflating our Type I error (i.e., the false positive rate), because we’ve already taken care of that up front by deliberately being overconservative in our analyses.

Unfortunately, while it’s true that our Type I error doesn’t suffer, the act of choosing which findings to report based on the results of a statistical test does have another unwelcome consequence. Specifically, there’s a very good chance that the effect sizes we end up reporting for statistically significant results will be artificially inflated–perhaps dramatically so.

Why would this happen? It’s actually entailed by the selection procedure. To see this, let’s take the classical measurement model, under which the variance in any measured variable reflects the sum of two components: the “true” scores (i.e., the scores we would get if our measurements were always completely accurate) and some random error. The error term can in turn be broken down into many more specific sources of error; but we’ll ignore that and just focus on one source of error–namely, sampling error. Sampling error refers to the fact that we can never select a perfectly representative group of subjects when we collect a sample; there’s always some (ideally small) way in which the sample characteristics differ from the population. This error term can artificially inflate an effect or artificially deflate it, and it can inflate or deflate it more or less, but it’s going to have an effect one way or the other. You can take that to the bank as sure as my name’s Bozo the Clown.

To put this in context, let’s go back to our BDI scores. Recall that what we observed is that clowns have higher BDI scores than non-clowns. But what we’re now saying is that that difference in scores is going to be affected by sampling error. That is, just by chance, we may have selected a group of clowns that are particularly depressed, or a group of non-clowns who are particularly jolly. Maybe if we could measure depression in all clowns and all non-clowns, we would actually find no difference between groups.

Now, if we allow that sampling error really is random, and that we’re not actively trying to pre-determine the outcome of our study by going out of our way to recruit The Great Depressed Mancini and his extended dysthymic clown family, then in theory we have no reason to think that sampling error is going to introduce any particular bias into our results. It’s true that the observed correlations in our sample may not be perfectly representative of the true correlations in the population; but that’s not a big deal so long as there’s no systematic bias (i.e., that we have no reason to think that our sample will systematically inflate correlations or deflate them). But here’s the problem: the act of choosing to report some correlations but not others on the basis of their statistical significance (or lack thereof) introduces precisely such a bias. The reason is that, when you go looking for correlations that are of a certain size or greater, you’re inevitably going to be more likely to select those correlations that happen to have been helped by chance than hurt by it.

Here’s a series of figures that should make the point even clearer. Let’s pretend for a moment that the truth of the matter is that there is in fact a positive correlation between clown status and all 10 depression measures. Except, we’ll make it 100 measures, because it’ll be easier to illustrate the point that way. Moreover, let’s suppose that the correlation is exactly the same for all 100 measures, at .3. Here’s what that would look like if we just plotted the correlations for all 100 measures, 1 through 100:

figure1

It’s just a horizontal red line, because all the individual correlations have the same value (0.3). So that’s not very exciting. But remember, these are the population correlations. They’re not what we’re going to observe in our sample of 20 clowns and 20 non-clowns, because depression scores in our sample aren’t a perfect representation of the population. There’s also error to worry about. And error–or at least, sampling error–is going to be greater for smaller samples than for bigger ones. (The reason for this can be expressed intuitively: other things being equal, the more observations you have, the more representative your sample must be of the population as a whole, because deviations in any given direction will tend to cancel each other out the more data you collect. And if you keep collecting, at the limit, your sample will constitute the whole population, and must therefore by definition be perfectly representative). With only 20 subjects in each group, our estimates of each group’s depression level are not going to be terrifically stable. You can see this in the following figure, which shows the results of a simulation on 100 different variables, assuming that all have an identical underlying correlation of .3:

figure2

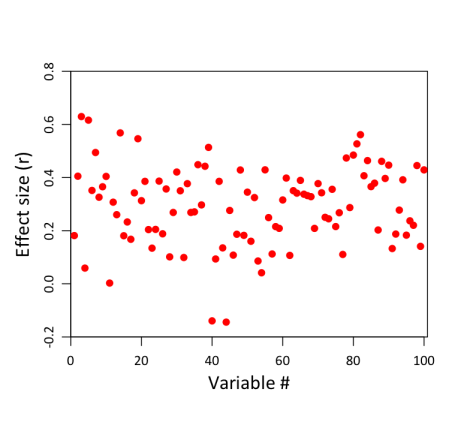
Notice how much variability there is in the correlations! The weakest correlation is actually negative, at -.18; the strongest is much larger than .3, at .63. (Caveat for more technical readers: this assumes that the above variables are completely independent, which in practice is unlikely to be true when dealing with 100 measures of the same construct.) So even though the true correlation is .3 in all cases, the magic of sampling will necessarily produce some values that are below .3, and some that are above .3. In some cases, the deviations will be substantial.

By now you can probably see where this is going. Here we have a distribution of effect sizes that to some extent may reflect underlying variability in population effect sizes, but is also almost certainly influenced by sampling error. And now we come along and decide that, hey, it doesn’t really make sense to report all 100 of these correlations in a paper; that’s too messy. Really, for the sake of brevity and clarity, we should only report those correlations that are in some sense more important and “real”. And we do that by calculating p-values and only reporting the results of tests that are significant at some predetermined level (in our case, p < .005). Well, here’s what that would look like:

figure3

This is exactly the same figure as the previous one, except we’ve now grayed out all the non-significant correlations. And in the process, we’ve made Bozo the Clown cry:

Why? Because unfortunately, the criterion that we’ve chosen is an extremely conservative one. In order to detect a significant difference in means between two groups of 20 subjects at p < .005, the observed correlation (depicted as the horizontal black line above) needs to be .42 or greater! That’s substantially larger than the actual population effect size of .3. Effects of this magnitude don’t occur very frequently in our sample; in fact, they only occur 16 times. As a result, we’re going to end up failing to detect 84 of 100 correlations, and will walk away thinking they’re null results–even though the truth is that, in the population, they’re actually all pretty strong, at .3. This quantity–the proportion of “real” effects that we’re likely to end up calling statistically significant given the constraints of our sample–is formally called statistical power. If you do a power analysis for a two-sample t-test on a correlation of .3 at p < .005, it turns out that power is only .17 (which is essentially what we see above; the slight discrepancy is due to chance). In other words, even when there are real and relatively strong associations between depression and clownhood, our sample would only identify those associations 17% of the time, on average.

That’s not good, obviously, but there’s more. Now the other shoe drops, because not only have we systematically missed out on most of the effects we’re interested in (in virtue of using small samples and overly conservative statistical thresholds), but notice what we’ve also done to the effect sizes of those correlations that we do end up identifying. What is in reality a .3 correlation spuriously appears, on average, as  a .51 correlation in the 16 tests that surpass our threshold. So, through the combined magic of low power and selection bias, we’ve turned what may in reality be a relatively diffuse association between two variables (say, clownhood and depression) into a seemingly selective and extremely strong association. After all the excitement about getting a high-profile publication, it might ultimately turn out that clowns aren’t really so depressed after all–it’s all an illusion induced by the sampling apparatus. So you might say that the clowns get the last laugh. Or that the joke’s on us. Or maybe just that this whole clown example is no longer funny and it’s now time for it to go bury itself in a hole somewhere.

Anyway, that, in a nutshell, was the point my commentary on the Vul et al paper made, and it’s the same point the Gelman and Wainer papers make too, in one way or another. While it’s a very general point that really applies in any domain where (a) power is less than 100% (which is just about always) and (b) there is some selection bias (which is also just about always), there were some considerations that were particularly applicable to fMRI research. The basic issue is that, in fMRI research, we often want to conduct analyses that span the entire brain, which means we’re usually faced with conducting many more statistical comparisons than researchers in other domains generally deal with (though not, say, molecular geneticists conducting genome-wide association studies). As a result, there is a very strong emphasis in imaging research on controlling Type I error rates by using very conservative statistical thresholds. You can agree or disagree with this general advice (for the record, I personally think there’s much too great an emphasis in imaging on Type I error, and not nearly enough emphasis on Type II error), but there’s no avoiding the fact that following it will tend to produce highly inflated significant effect sizes, because in the act of reducing p-value thresholds, we’re also driving down power dramatically, and making the selection bias more powerful.

While it’d be nice if there was an easy fix for this problem, there really isn’t one. In behavioral domains, there’s often a relatively simple prescription: report all effect sizes, both significant and non-significant. This doesn’t entirely solve the problem, because people are still likely to overemphasize statistically significant results relative to non-significant ones; but at least at that point you can say you’ve done what you can. In the fMRI literature, this course of action isn’t really available, because most journal editors are not going to be very happy with you when you send them a 25-page table that reports effect sizes and p-values for each of the 100,000 voxels you tested. So we’re forced adopt other strategies. The one I’ve argued for most strongly is to increase sample size (which increases power and decreases the uncertainty of resulting estimates). But that’s understandably difficult in a field where scanning each additional subject can cost $1,000 or more. There are a number of other things you can do, but I won’t talk about them much here, partly because this is already much too long a post, but mostly because I’m currently working on a paper that discusses this problem, and potential solutions, in much more detail.

So now finally I get to the Ioannidis article. As I said, the basic point is the same one made in my paper and Gelman’s and others, and the one I’ve described above in excruciating clownish detail. But there are a number of things about the Ioannidis that are particularly nice. One is that Ioannidis considers not only inflation due to selection of statistically significant results coupled with low power, but also inflation due to the use of flexible analyses (or, as he puts it, “vibration” of effects–also known as massaging the data). Another is that he considers cultural aspects of the phenomenon, e.g., the fact that investigators tend to be rewarded for reporting large effects, even if they subsequently fail to replicate. He also discusses conditions under which you might actually get deflation of effect sizes–something I didn’t touch on in my commentary, and hadn’t really thought about. Finally, he makes some interesting recommendations for minimizing effect size inflation. Whereas my commentary focused primarily on concrete steps researchers could take in individual studies to encourage clearer evaluation of results (e.g., reporting confidence intervals, including power calculations, etc.), Ioannidis focuses on longer-term solutions and the possibility that we’ll need to dramatically change the way we do science (at least in some fields).

Anyway, this whole issue of inflated effect sizes is a critical one to appreciate if you do any kind of social or biomedical science research, because it almost certainly affects your findings on a regular basis, and has all sorts of implications for what kind of research we conduct and how we interpret our findings. (To give just one trivial example, if you’ve ever been tempted to attribute your failure to replicate a previous finding to some minute experimental difference between studies, you should seriously consider the possibility that the original effect size may have been grossly inflated, and that your own study consequently has insufficient power to replicate the effect.) If you only have time to read one article that deals with this issue, read the Ioannidis paper. And remember it when you write your next Discussion section. Bozo the Clown will thank you for it.

Ioannidis, J. (2008). Why Most Discovered True Associations Are Inflated Epidemiology, 19 (5), 640-648 DOI: 10.1097/EDE.0b013e31818131e7

Yarkoni, T. (2009). Big Correlations in Little Studies: Inflated fMRI Correlations Reflect Low Statistical Power-Commentary on Vul et al. (2009) Perspectives on Psychological Science, 4 (3), 294-298 DOI: 10.1111/j.1745-6924.2009.01127.x